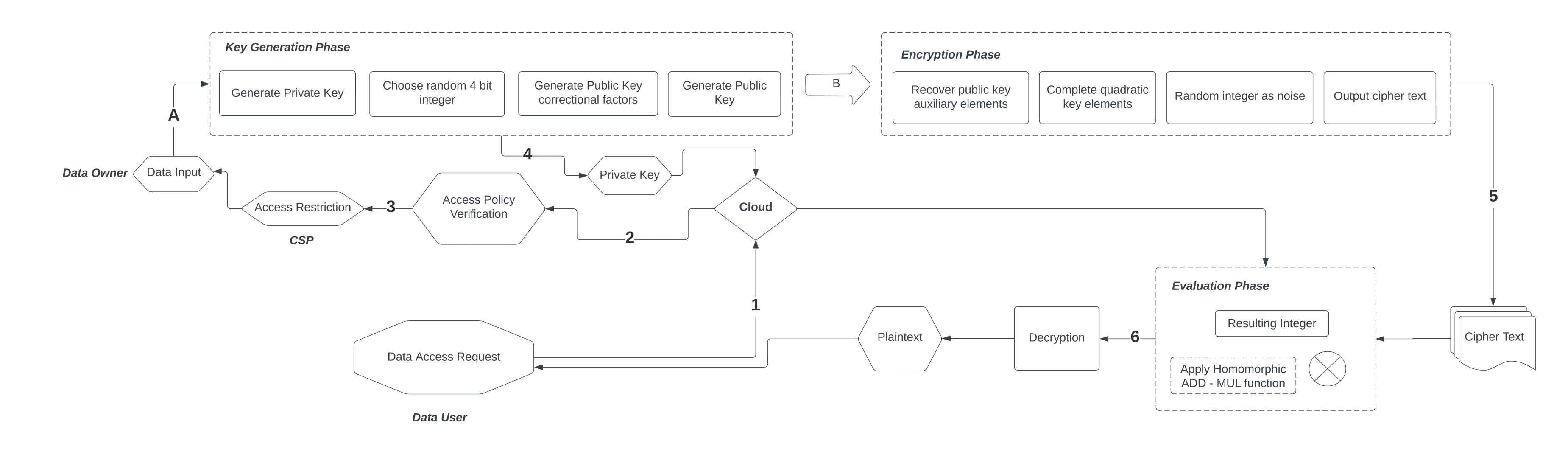
Upcoming features and milestones for 2024 →

Hidden in plain sight.

Enabling privacy-preserving quantitative analysis on sensitive financial data sets without exposing underlying transaction information.

View Our Finances Our Impact

Quantum-Proof.

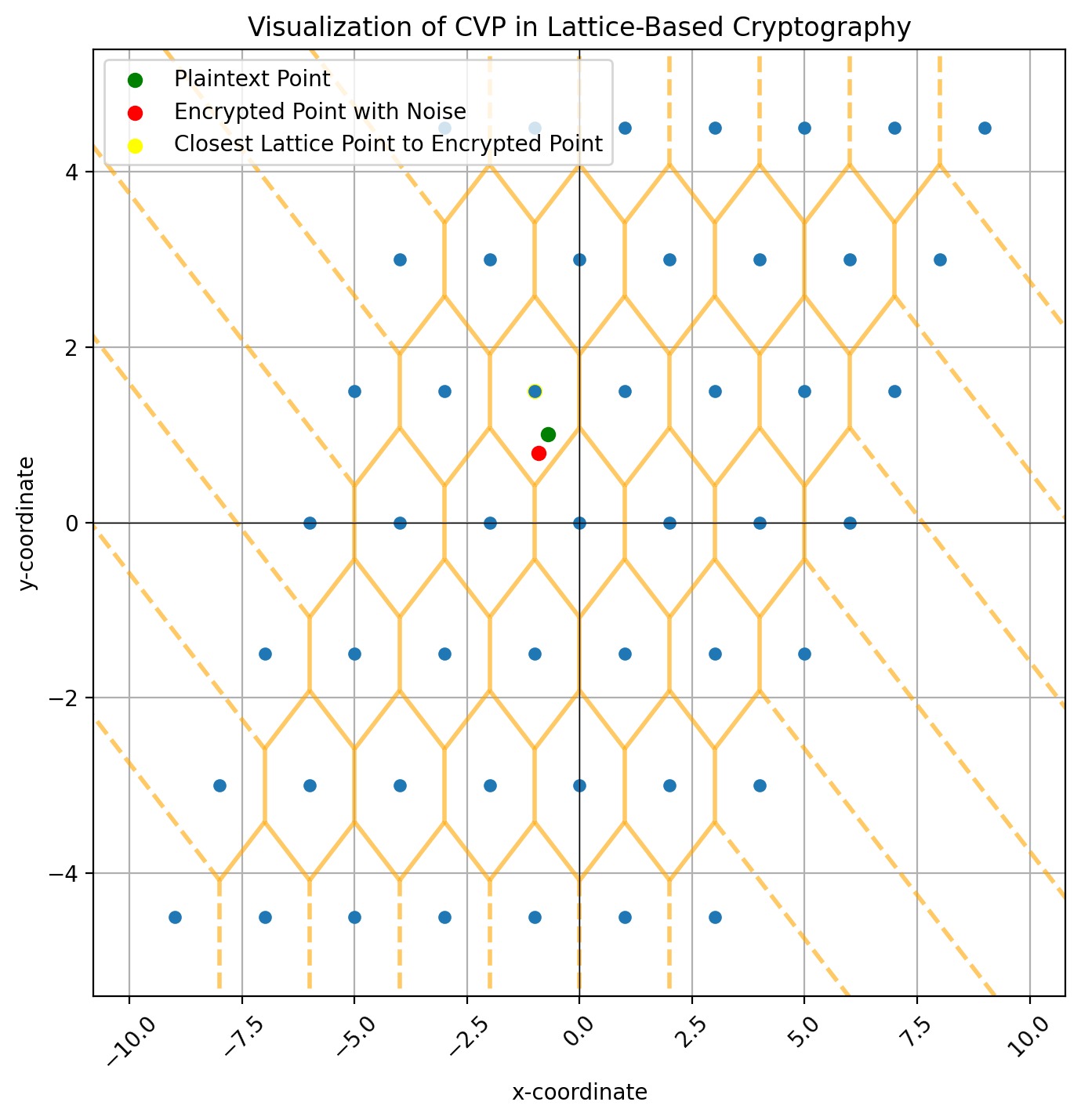
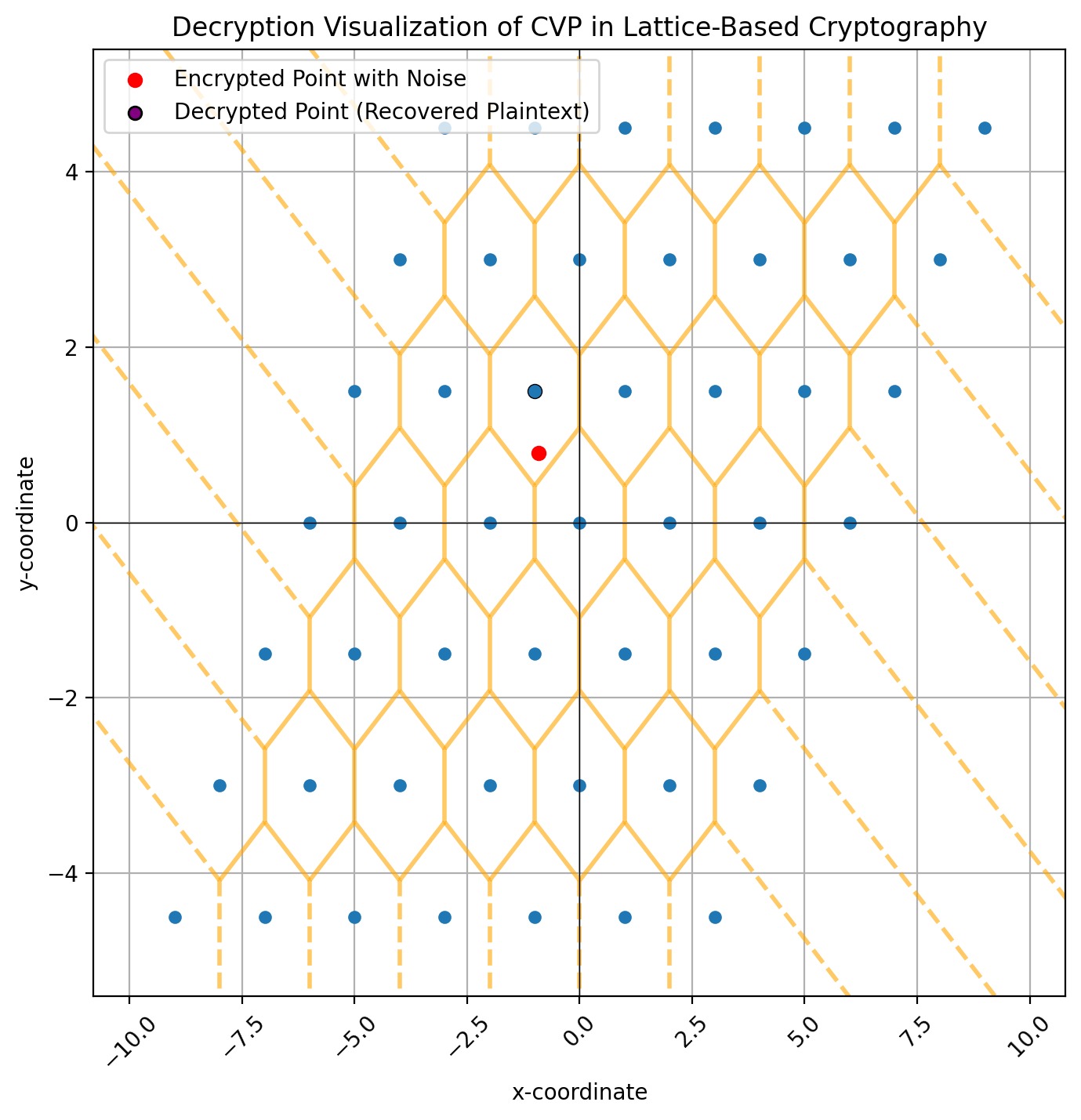
Future proof lattice-based cryptography in a changing quantum landscape.

Easy to adopt.
Seamlessly integrates with existing data infrastructure so that your organization can future-proof sensitive data quickly.
High performance at scale.
Uncompromising speed, even on massive datasets. Computations execute efficiently with minimal overhead.
Compliance-Friendly.
Adheres to data privacy regulations including HIPAA, GDPR, CCPA, and more.
Product screenshot Product screenshot Product screenshot

Progress secured within.

The future waits for no one – financial services demand every edge yet data risks obstruct growth. Until now.

Homomorphic
Our encryption enables computations on ciphertexts - mathematical operations execute directly on encrypted data without decryption.
Lattice-based
Lattices enable homomorphism. This approach fuels the privacy-preserving data analysis capabilities within our solution.
Flexible
Customizable encryption schemes, depth of homomorphic operations, and transparent controls around access and auditing.
Accurate
Computations on encrypted data produce results identical to operations performed on unencrypted plaintext data.

How it works

The programmer defines 2 or more vectors, called the bases. These bases form a lattice. A plaintext point is plotted on the xy plane, and it is given a controlled amount of noise that distorts the coordinates of the plaintext point, thus encrypting it. Decoding this can only be done with the private key, and because of the nature of lattice encryption, hacking even with quantum computers is nearly impossible, as some lattices can extend infinitely and have multiple dimensions. Two common lattice based problems are CVP and SVP. Closest Vector problem asks to find the lattice point closest to the encrypted data point, to determine the position of the plaintext point. These problems are extremely computationally complex, meaning algorithms based on these problems are resistant to attacks by quantum computers.

Flowchart